
For images with space as its physical dimension, the Fourier transform is in spatial frequency. The Fourier transform is a linear transform that transform a physical dimension to its inverse. I used Scilab 5.5.2 with the SIVP package. In this blog post, I’ll explore the Fourier transform and its use in image processing. Back then, I thought that was pretty cool. Pure tones of frequencies corresponding to the peaks in the FT are combined to produce the sound. For example, we simulated the sound of a musical instrument by sampling the sound of the aforementioned instrument and taking the Fourier transform of the sample sound. During these classes, we mostly used the Fourier transform for processing sound signals. I first encountered the Fourier transform in my Applied Physics 183 and Applied Physics 185 classes. results can be accessed from f_peaks.Exploring the Fourier transform in imaging processing Peaks are detected and ordered in descending orderĦ. The amplitude of the complex number calculated,ĥ. Input data ordered as per generated order,Ĥ.
Scilab fft code#
Code generates a bit reversed the order for the given sample size (details on bit reversing on references: step 2)Ģ. byte sine_data = //imaginory part of transformġ. Where f_peaks is the most dominant frequency and further values in descending order. After every run of FFT function this array updates. The sine_data needs to paste at top of code to declare it as a global variable.Īpart from sine_data, an array called f_peaks declared as a global variable. Doing so will eliminate the need of using storing numbers as float and we can store it as byte which takes 1/4th space on Arduino. To counter this issue value of sine for 0 to 90 degrees is stored as multiple of 255. Which makes code significantly slower (doubles time for 64 samples). The inbuilt function of Arduino is not fast enough and takes a good amount of time to provide the required value. Mathematically it is shown that for N number of samples it takes N*N complex multiplication.Ĭalculation FFT takes the value of various sine and cosine multiple times. Such intense calculation takes a higher time. Here a signal is tested with 5 frequencies, so signal needs to be multiplied by five frequencies. However for the value, f2 and f5 output won't be zero, but significantly higher than the rest of the values.

So if our signal is multiplied by f1 summation of multiplication will be zero (near to zero for real application).
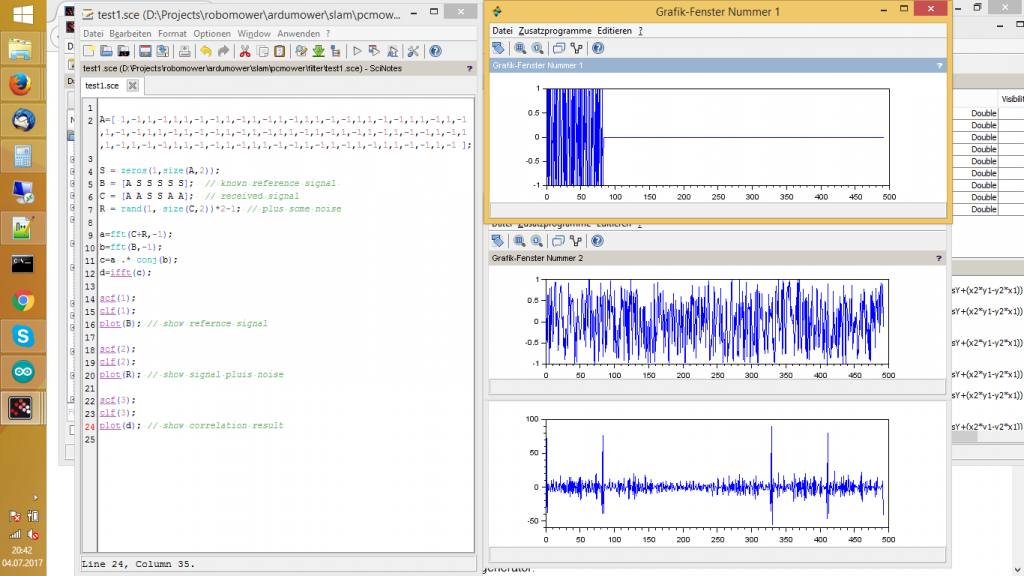
In our case, If these two multiplication frequency has the same (or very close) frequency that sum of multiplication is the nonzero number. It can be shown mathematically that -summation of multiplication of two harmonic data-set having different frequency tends to zero(higher number of data can lead to batter result). This signal is multiplied by test sine waves of values f1 to f5. In the image, a signal is shown which is a combination of two frequencies f2 and f5. These methods are extremely slow for any real-time application. I tried to explain the working of DFT(discrete Fourier transform)in one of the previous instructable (. So any time-based signal can be also shown as a combination of the various sine of different amplitudes. Any signal can be composed of a combination of various sinusoidal waves.


 0 kommentar(er)
0 kommentar(er)
